Though we’re not fans of the CAPM definition of risk, the framework is widely known and serves as a useful reference point for discussing some very common questions.  In this post we’ll look at the cost of capital for a convertible bond from a number of perspectives, including intuitive/qualitative (which provides quick directional answers) and a more precise numerical calculation.

A Review of Basic WACC Tradeoffs

Recall that in the Modigliani-Miller WACC framework, an asset’s (equivalently, a company’s) cost of capital is determined by two parameters:

1. asset risk
2. funding tax shield

Changes to a company’s capital structure (assuming no change to asset risk) only affects WACC to the extent that the firm’s tax shield changes. The following capital structure changes increase tax shield, and thus decrease WACC:

1. Replacing equity with debt (larger interest expense produces greater tax shield)
2. Increasing marginal tax rate
3. Increasing debt coupon

The last point seems counterintuitive:  as the cost of debt rises, WACC falls.  What’s going on?  Recall that the total asset risk is divided between lenders and shareholders.  If the cost of debt rises, this implies that lenders are shouldering more of the asset risk; to keep the total asset risk constant, we find shareholders bearing less asset risk, so the cost of equity must fall.  Ignoring taxes, these two effects perfectly cancel, leaving WACC unchanged; including the greater tax shield (from the higher interest expense) leaves WACC lower.

 

How Convertible Debt Changes WACC

Keeping in mind that capital structure changes affect WACC only through their impact on tax shield, it’s easy to answer some basic cost-of-capital questions regarding convertible debt. We assume that, compared to a company’s straight debt, convertible debt carries a lower cash coupon, and coupon payments are tax deductible.

Convertible Debt Costs More Than Straight Debt

If the convertible bond replaces senior debt, then the company’s interest expense falls, the tax shield falls, and WACC rises.

• If the convertible bond is subordinated to the firm’s senior debt, then the yield on the remaining senior debt should slightly fall, which further decreases interest expense and tax shield, further increasing WACC.
• Cost of equity (for the existing common shares) should be unchanged (except for a small secondary effect, where the reduced tax shield slightly reduces market capitalization)

Convertible Debt Costs Less Than Equity

If the convertible bond replaces equity, then the company’s interest expense rises, the tax shield rises, and WACC falls.

•  Cost of (senior) debt is unchanged
• The impact on the cost of common equity is complex.  The convertible replaces some of the original equity, so there’s less total equity in the capital structure.  Note also the original shareholders’ claim on enterprise value is diluted by the presence of the convertible bond in the capital stack, but the amount of dilution depends on the price of the stock:  as price increases, conversion (and original shareholder dilution) likelihood increases.

This last point deserves one additional exercise.  If we think about the convertible bond as a combination of straight debt plus a warrant, and replace corresponding portions of the original capital structure’s debt and equity with the convertible bond, then we end up with a capital structure stack as follows:

1. remaining (senior) debt
2. debt component of convertible
3. warrant component of convertible
4. remaining common equity

Ignoring tax shield changes, the value of 1+2 is the same as the original debt; the value of 3+4 is the same as the original equity.  What happens to the cost of “equity” (components 3 and 4)?  We have the same fraction of equity in the capital structure as before, so we expect equity risk (and thus cost of equity) to be unchanged.  However the fraction of equity value subsumed by the warrant changes with the value of equity:  the higher the equity value, the greater the fraction of equity claimed by the warrant holders.  In this way the convertible bond’s warrant component acts to dampen the effect of enterprise value swings for the common equity holders.  This means that the beta of common equity has fallen; to keep overall equity cost&risk the same, the beta of the warrant must be greater than the beta of the original equity.

 

WACC of a Convertible Bond

We can focus more narrowly on the cost of the convertible bond itself.  Two common approaches:

1. Assuming the capital structure changes from D+E to D+C+E (debt + convertible + equity), we can calculate the change on the company’s overall WACC (based on change in tax shield), calculate the updated costs of capital for D and E after the introduction of the convertible (following the reasoning above), and solve for the cost of the convertible.
2. We can calculate the cost of capital for the convertible directly; a common technique bifurcates the convertible bond into a straight debt component and a warrant component.

In this section we’ll derive the latter.  The steps in the process are as follows:

1. Begin with market price for the convertible debt, and pricing information for the company’s debt and equity
2. Calculate the values of the convertible’s debt and warrant components
3. Calculate costs of capital for the debt and warrant components
4. Calculate a weighted average cost of capital for the convertible

The most difficult step is calculating the cost of the warrant; fortunately there is a straightforward way to calculate the beta of a warrant from the properties of the underlier.

 

Bifurcate the Convertible Bond

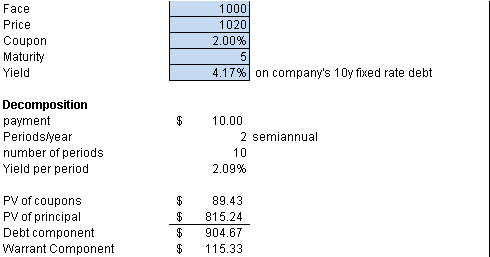
From the convertible’s term sheet and pricing information, we can calculate the value of its straight debt component by discounting the coupons and principle flows at the cost of debt.  We can solve for the value of the warrant component as a plug:

Note that the convertible carries a coupon (2%) markedly less than the cost of debt (4.17%).

 

Find the Cost of the Warrant

If we can find the beta of the warrant, we can use CAPM to price the warrant.  Fortunately standard option pricing theory yields a simple formula for an option’s beta as a function of the underlier:

     Beta_call = Beta_stock * Elasticity_call

where

Elasticity_call = (S/C) * delta

That is, the beta of the call is the delta of the option times the beta of the underlier, and we have to normalize for the different prices of the instruments by multiplying by S/C (prices of stock and call respectively).  (See  http://groups.yahoo.com/neo/groups/OptionClub/conversations/topics/5516?var=1 for more discussion.)

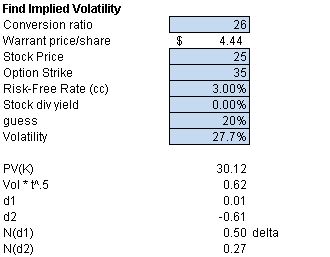
We know the prices of the stock and the call (warrant); all we need is the delta of the warrant.  We can derive this by using the Black-Scholes formula, which produces the delta of the option as an intermediate result.  B-S requires the volatility of the underlier, which we can impute by using B-S against the price of the warrant component of the convertible bond.   The calculations are as follows:

Here we used a numerical technique to solve for the implied volatility (27.7%), and then calculated delta using B-S.

 

Calculate WACC of the Convertible Bond

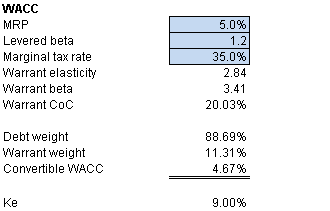
With the warrant’s delta in hand, we can calculate beta of the warrant (given beta of the underlier, which is the common equity in this case); the cost of capital for the warrant; and the WACC of the convertible bond overall:

As expected, the cost of the convertible bond (4.67%) is greater than the cost of debt (4.17%) and less than the cost of equity (9.0%).  Note also that the warrant beta (3.4) is almost three times the stock beta (1.2).  However the relatively small weight of the warrant in the overall convertible leaves the convertible still costing less than equity.

The following chart shows various components’ cost of capital as a function of stock price:

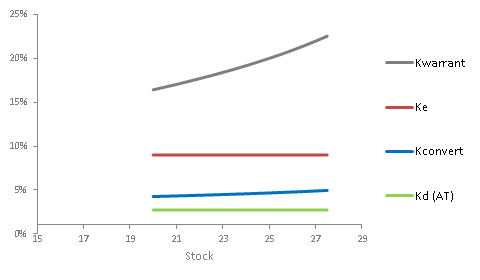(Note that in this sensitization analysis we have changed only the stock price; we haven’t changed the market price of the convertible bond.)  Under these assumptions, as stock price increases, we find the cost of capital for the warrant increasing markedly, which pushes the overall cost of the convertible upward.